Page 123 - The Indian Optician Digital Edition March-April 2024
P. 123
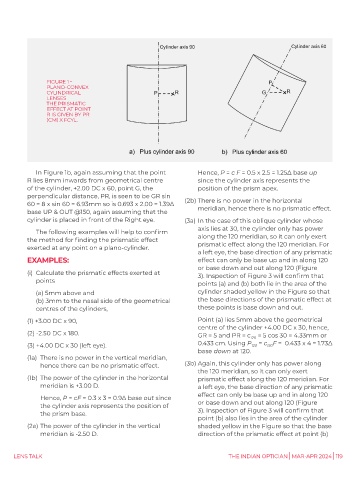
FIGURE 1 -
PLANO-CONVEX
CYLINDRICAL
LENSES
THE PRISMATIC
EFFECT AT POINT
R IS GIVEN BY PR
(CM) X FCYL.
In Figure 1b, again assuming that the point Hence, P = c F = 0.5 x 2.5 = 1.25Δ base up
R lies 8mm inwards from geometrical centre since the cylinder axis represents the
of the cylinder, +2.00 DC x 60, point G, the position of the prism apex.
perpendicular distance, PR, is seen to be GR sin
60 = 8 x sin 60 = 6.93mm so is 0.693 x 2.00 = 1.39∆ (2b) There is no power in the horizontal
base UP & OUT @150, again assuming that the meridian, hence there is no prismatic effect.
cylinder is placed in front of the Right eye. (3a) In the case of this oblique cylinder whose
axis lies at 30, the cylinder only has power
The following examples will help to confirm
the method for finding the prismatic effect along the 120 meridian, so it can only exert
exerted at any point on a plano-cylinder. prismatic effect along the 120 meridian. For
a left eye, the base direction of any prismatic
EXAMPLES: effect can only be base up and in along 120
or base down and out along 120 (Figure
(i) Calculate the prismatic effects exerted at 3). Inspection of Figure 3 will confirm that
points points (a) and (b) both lie in the area of the
(a) 5mm above and cylinder shaded yellow in the Figure so that
(b) 3mm to the nasal side of the geometrical the base directions of the prismatic effect at
centres of the cylinders, these points is base down and out.
(1) +3.00 DC x 90, Point (a) lies 5mm above the geometrical
centre of the cylinder +4.00 DC x 30, hence,
(2) -2.50 DC x 180. GR = 5 and PR = c = 5 cos 30 = 4.33mm or
120
(3) +4.00 DC x 30 (left eye). 0.433 cm. Using P = c F = 0.433 x 4 = 1.73Δ
120
120
base down at 120.
(1a) There is no power in the vertical meridian,
hence there can be no prismatic effect. (3b) Again, this cylinder only has power along
the 120 meridian, so it can only exert
(1b) The power of the cylinder in the horizontal prismatic effect along the 120 meridian. For
meridian is +3.00 D. a left eye, the base direction of any prismatic
effect can only be base up and in along 120
Hence, P = cF = 0.3 x 3 = 0.9Δ base out since
the cylinder axis represents the position of or base down and out along 120 (Figure
the prism base. 3). Inspection of Figure 3 will confirm that
point (b) also lies in the area of the cylinder
(2a) The power of the cylinder in the vertical shaded yellow in the Figure so that the base
meridian is -2.50 D. direction of the prismatic effect at point (b)
LENS TALK THE INDIAN OPTICIAN | MAR-APR 2024 | 119